Exploring Inverse Trigonometric Ratios: Unveiling the Mysteries of Angle Relationships:

Introduction:

Trigonometry, the study of triangles and the relationships between their sides and angles, is a cornerstone of mathematics with applications spanning from physics and engineering to architecture and navigation. Among the fundamental concepts within trigonometry are trigonometric ratios, such as sine, cosine, and tangent, which relate the angles of a right triangle to the lengths of its sides.

While these ratios are invaluable tools for solving problems involving right triangles, they're not without limitations. Enter the inverse trigonometric ratios, an intriguing extension that allows us to work backward, finding angles when the side lengths are known. In this blog post, we'll delve into the fascinating realm of inverse trigonometric functions, exploring their definitions, properties, and real-world applications.

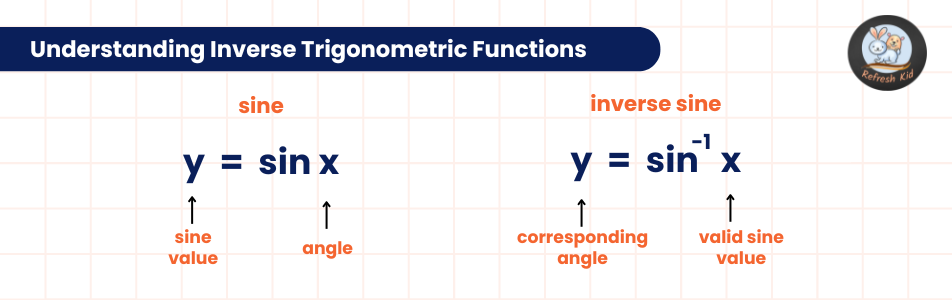
Understanding Inverse Trigonometric Functions:

Inverse trigonometric functions, denoted as $\sin^{-1}(x) \), \( \cos^{-1}(x)$ , and $\tan^{-1}(x)$ , among others, are the counterparts of the standard trigonometric functions sine, cosine, and tangent. Rather than mapping angles to ratios of sides, these functions map ratios back to angles. In essence, they help us answer the question: "What angle corresponds to a given ratio?"

For example, if we know the ratio of the opposite side to the hypotenuse of a right triangle, we can use the inverse sine function to find the angle opposite that side. This process is invaluable in various fields where angles need to be determined from known side lengths.

Inverse Trigonometric Functions - W3schools

Example:

Let's take a simple example involving inverse trigonometric ratios. Consider finding the angle θ in a right triangle given the side lengths.

Let's say we have a right triangle with sides:

Adjacent side (side adjacent to the angle we want to find): 4 units
Hypotenuse (longest side, opposite the right angle): 5 units

To find the angle θ, we'll use the inverse trigonometric ratio. Since we have the adjacent side and the hypotenuse, we'll use the inverse cosine function (arccosine) since cosine relates the adjacent side to the hypotenuse.

So, $\Theta = cos^{-1}\frac{4}{5}$

Using a calculator or any tool that provides trigonometric functions, we find:

θ $\approx cos^{-1}0.8$ $\approx$ 36.87 degrees.

So, in this case, the angle θ is approximately 36.87 degrees.

Properties of Inverse Trigonometric Functions:

1. Domain and Range: Unlike their standard counterparts, inverse trigonometric functions have restricted domains and ranges to ensure they are one-to-one functions, allowing for unambiguous inverse operations. For instance, the domain of $\sin^{-1}(x)$ is $[-1, 1]$ , and its range is $$[- \frac{\pi}{2}$ , $\frac{\pi}{2}]$$ in radians or $[-90^\circ, 90^\circ]$ in degrees.

2. Symmetry: The graphs of inverse trigonometric functions exhibit symmetry about the line y = x. This symmetry reflects the inverse relationship between angles and ratios.

3. Principal Values: Inverse trigonometric functions have multiple possible angles for a given ratio. To ensure uniqueness, mathematicians define principal values within specific ranges. For instance, the principal value of $\sin^{-1}(0.5)$ is $\frac{\pi}{6}$ radians or $30^\circ$ .

Real-World Applications:

1. Engineering: In civil and mechanical engineering, inverse trigonometric functions are used to design structures, calculate forces, and determine angles in various mechanical systems.

2. Physics: In physics, particularly in mechanics and waves, inverse trigonometric functions help analyze oscillatory motion, harmonic functions, and waveforms.

3. Navigation: In navigation and geolocation, inverse trigonometric functions play a crucial role in determining positions based on satellite signals or celestial observations.

4. Computer Graphics: In computer graphics and game development, inverse trigonometric functions are utilized to calculate angles for animations, camera movements, and collision detection.

Conclusion:

Inverse trigonometric functions are powerful tools that unlock a deeper understanding of angle relationships within right triangles. By allowing us to find angles from known side lengths, they enable precise calculations in various fields ranging from engineering and physics to computer science and beyond.

As we continue to explore the intricate interplay between angles and ratios, the significance of inverse trigonometric functions becomes ever more apparent, serving as indispensable assets in our mathematical toolkit.

In conclusion, while the world of trigonometry may seem daunting at first, delving into inverse trigonometric functions reveals a fascinating landscape of connections and applications, enriching our comprehension of the fundamental principles that govern the world around us.