Volume of Cones

Understanding the Volume of Cones: Practical Guide with Numerical Examples:

Introduction:

Geometry is a fundamental branch of mathematics that extends beyond theoretical concepts into everyday practical applications. One such concept is the volume of a cone, an essential topic for students and professionals in various fields such as architecture, engineering, and mathematics. This guide will walk you through the process of calculating the volume of cones, complete with numerical examples to enhance your understanding.

What is a Cone?

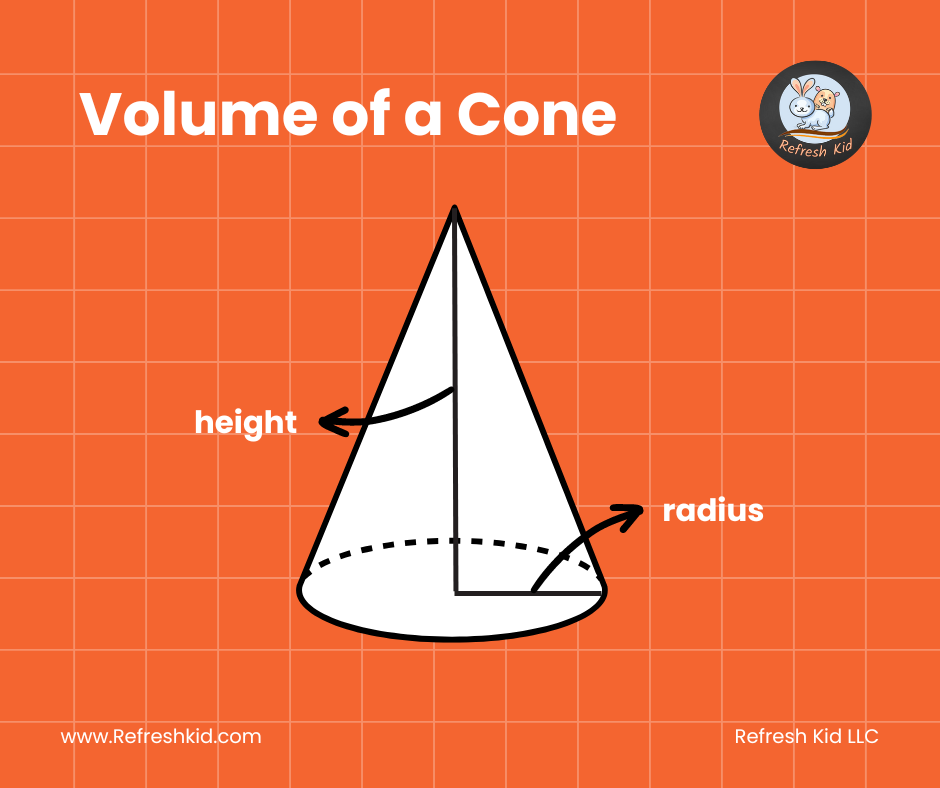
A cone is a three-dimensional geometric shape that tapers smoothly from a flat, circular base to a point called the apex or vertex. Cones are classified into two types: right circular cones, where the apex is directly above the center of the base, and oblique cones, where the apex is not aligned with the center of the base.

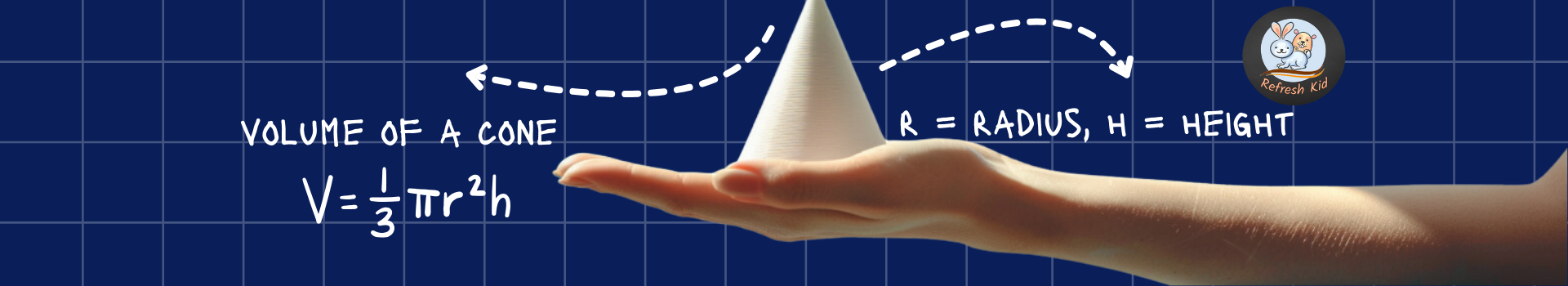
Formula for Calculating the Volume of a Cone:

The volume of a cone can be calculated using the formula: where:

• (pi) is a constant approximately equal to 3.14159.
•  is the radius of the circular base of the cone.
• is the height of the cone, measured from the base to the apex.

Numerical Examples:

Example 1: Calculating the Volume of a Right Circular Cone

Suppose you have a right circular cone with a radius of 3 cm and a height of 5 cm. Plugging these values into the formula, the volume is calculated as follows:  cubic cm This example demonstrates how to compute the volume for a cone with given dimensions, a vital skill in fields requiring geometric calculations.

Example 2: Real-World Application in Engineering

Consider an engineering project that requires a conical funnel with a diameter of 10 cm and a height of 15 cm. First, calculate the radius (half of the diameter): cm Using the volume formula: cubic cm This example illustrates the application of the cone volume formula in designing components with specific volume requirements.

Example 3: Smaller Cone Dimensions

Imagine you are given a cone with a radius of 2 inches and a height of 6 inches. Applying the volume formula:  cubic inches. This example shows the calculation for a smaller cone, often useful in crafting or model building.

Example 4: A Cone with Large Dimensions

Consider a large decorative cone used in a public art installation with a radius of 20 feet and a height of 50 feet. The volume calculation would be: cubic feet. This scenario is typical in architectural designs and large-scale constructions.

Example 5: Cone with Decimal Measurements

Suppose a cone used in a laboratory apparatus has a radius of 4.5 cm and a height of 12.5 cm. The volume is:  cubic cm. Precision is crucial in laboratory settings where exact volumes are necessary.

Real-Life Examples of Cone Applications:

Ice Cream Cones:

One of the most relatable uses of cones is in ice cream cones, where understanding volume helps businesses determine how much ice cream fits into different cone sizes, optimizing serving sizes and pricing.

Traffic Cones:

Traffic cones, essential for road safety and management, need specific volumes for stability and effectiveness in various weather conditions.

Conical Flasks in Laboratories:

In chemistry, conical flasks are used to mix or store solutions. The volume of these flasks is crucial for precise scientific experiments and results.

Conical Towers and Roofs:

Architectural designs often incorporate conical towers and roofs, particularly in historical and modern artistic structures, where the volume calculations assist in stability and aesthetic specifications.

Party Hats:

Party hats, typically cone-shaped, provide a fun example of how cone calculations can be applied in designing festive supplies, ensuring the hat's material is used efficiently.

Conclusion:

Understanding how to calculate the volume of cones is crucial for anyone involved in fields that utilize geometric shapes. By mastering this formula, you can enhance your mathematical skills and apply them to solve real-world problems. Whether you are a student, teacher, or professional, the ability to compute the volume of cones is an invaluable skill.