Surface Area of Pyramids

Apr 20 , 2024
By Refreshkid LLC

Comprehensive Guide to Calculating Surface Area of Pyramids:

Introduction to Surface Area of Pyramids:

Understanding the surface area of geometric shapes is fundamental in mathematics, especially for those involved in fields such as architecture, engineering, and design. Pyramids, with their distinctive base and triangular sides, present a fascinating challenge in this area. This blog post provides a detailed exploration of how to calculate the surface area of pyramids, complete with numerical examples that make learning this concept straightforward and engaging.

What is a Pyramid?

A pyramid is a polyhedron formed by connecting a polygonal base and a point, called the apex, which is not in the same plane as the base. Pyramids are named according to the shape of their base, such as triangular pyramids, square pyramids, and so on.

Types of Surface Areas in Pyramids:

Calculating the surface area of a pyramid involves determining the total area of all the surfaces that make up the pyramid.

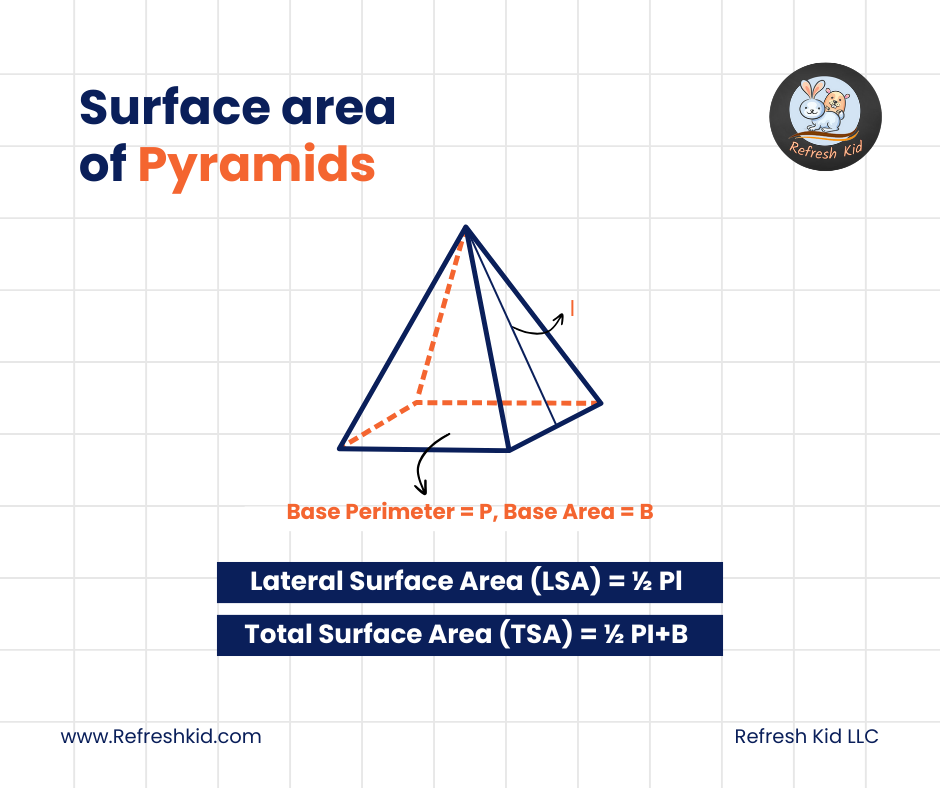
Lateral Surface Area (LSA):

The lateral surface area of a pyramid is the total area of all the triangular faces, excluding the base. The formula for the lateral surface area depends on the type of pyramid and involves the slant heights of the sides.

Total Surface Area (TSA):

The total surface area of a pyramid is the sum of its lateral surface area and the area of its base. This is the total exterior surface area of the pyramid.

Formulas for Surface Area of Pyramids:

Formula for the Surface Area of a Square Pyramid:
For a square pyramid, which has a square base:

Lateral Surface Area: $\text{LSA} = 2 \times \text{Base Length} \times \text{Slant Height}$
Total Surface Area: $\text{TSA} = \text{LSA} + \text{Base Area}$

Formula for the Surface Area of a Triangular Pyramid:
For a triangular pyramid, which has a triangular base:

Lateral Surface Area: $\text{LSA} = \frac{1}{2} \times \text{Perimeter of Base} \times \text{Slant Height}$
Total Surface Area: $\text{TSA} = \text{LSA} + \text{Base Area}$

Numerical Examples:

Example 1:

Suppose you have a square pyramid with a base side length of 6 meters and a slant height of 7 meters.

Base Area: $6 \times 6 = 36 \text{ m}^2$
Lateral Surface Area: $2 \times 6 \times 7 = 84 \text{ m}^2$
Total Surface Area: $84 + 36 = 120 \text{ m}^2$

Example 2:

Consider a triangular pyramid where each side of the triangular base is 4 meters, and the slant height is 5 meters.

Base Area (assuming equilateral triangle): $\sqrt{3}/4 \times 4^2 = 4\sqrt{3} \text{ m}^2$
Lateral Surface Area: $\frac{1}{2} \times 12 \times 5 = 30 \text{ m}^2$
Total Surface Area: $30 + 4\sqrt{3} \approx 36.93 \text{ m}^2$

Example 3:

Consider a pyramid with a rectangular base where the length of the base is 8 meters and the width is 5 meters. Assume a slant height of 10 meters for the sides involving the length and 7 meters for the sides involving the width.

Base Area: $8 \times 5 = 40 \text{ m}^2$
Lateral Surface Area: For the sides with the length of 8 meters,
$2 \times (8 \times 10) / 2 = 80 \text{ m}^2$
And for the sides with the width of 5 meters,
$2 \times (5 \times 7) / 2 = 35 \text{ m}^2$
Total LSA = $80 + 35 = 115 \text{ m}^2$
Total Surface Area: $\$ 115 + 40 = 155 \text{ m}^2 $$

Example 4:

Assume a pyramid with a regular pentagonal base where each side of the base is 3 meters, and the slant height from each side to the apex is 6 meters.

Base Area: Using the formula for the area of a regular pentagon, $\text{Area} = \frac{1}{4} \sqrt{5(5 + 2\sqrt{5})} \times \text{side length}^2$ ,
$\text{Base Area} = \frac{1}{4} \sqrt{5(5 + 2\sqrt{5})} \times 3^2 \approx 15.48 \text{ m}^2$
Lateral Surface Area: Each triangular side panel can be calculated as,
$\frac{1}{2} \times 3 \times 6 = 9 \text{ m}^2$
Total LSA = $9 \times 5 = 45 \text{ m}^2$
Total Surface Area: $45 + 15.48 \approx 60.48 \text{ m}^2$

Example 5:

Suppose a pyramid with an octagonal base where each side of the base is 2 meters and the slant height from each side to the apex is 9 meters.

Base Area: Using the formula for the area of a regular octagon, $\text{Area} = 2(1 + \sqrt{2}) \times \text{side length}^2$ ,
$\text{Base Area} = 2(1 + \sqrt{2}) \times 2^2 \approx 16.97 \text{ m}^2$
Lateral Surface Area: Each triangular side panel can be calculated as,
$\frac{1}{2} \times 2 \times 9 = 9 \text{ m}^2$
Total LSA = $9 \times 8 = 72 \text{ m}^2$

Total Surface Area: $72 + 16.97 \approx 88.97 \text{ m}^2$

These examples demonstrate how to calculate the surface area for pyramids with different base shapes and should help students and professionals alike in understanding the geometric concepts and their applications in real-world scenarios.

Conclusion:

Calculating the surface area of pyramids can enhance your understanding of geometry and is essential for various practical applications. By mastering these formulas and practicing through examples, students and educators can improve their mathematical skills and confidence in dealing with complex geometric figures.

For more educational content and tutorials in mathematics, continue exploring our website. Whether you're preparing for an exam or undertaking a project that involves geometric calculations, our resources can help you succeed.

Surface Area of Pyramids

Comprehensive Guide to Calculating Surface Area of Pyramids:

Introduction to Surface Area of Pyramids:

What is a Pyramid?

Types of Surface Areas in Pyramids:

Lateral Surface Area (LSA):

Total Surface Area (TSA):

Formulas for Surface Area of Pyramids:

Numerical Examples:

Example 1:

Example 2:

Example 3:

Example 4:

Example 5:

Conclusion:

Trending Articles

Sample Space and Representation of a Sample Space (List, Table or Tree Diagram)

Volume of Pyramids

Volume of Spheres and Hemispheres

Volume of Cones

Volume of cylinders

Categories List

Our Best Services

Math Tutoring

Counseling

Admissions

Science Help

College Prep

You can be your own Guiding star with our help