Volume of Spheres and Hemispheres

Mastering the Volume of Spheres and Hemispheres: A Detailed Guide with Examples:

Introduction:

Geometry not only shapes our understanding of the world but also underpins many scientific and practical endeavors. Among the fundamental concepts in geometry is the volume of spheres and hemispheres. This guide provides a thorough exploration of how to calculate these volumes, supported by clear numerical examples that are useful in academic and practical contexts.

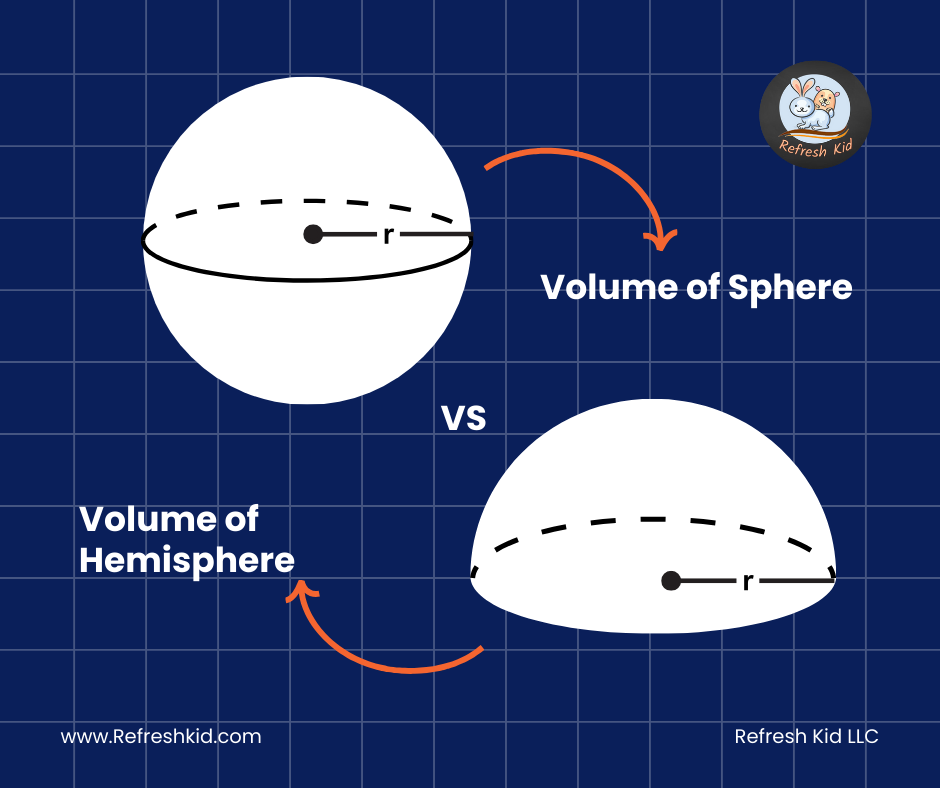
What is a Sphere?

A sphere is a perfectly round geometrical object in three-dimensional space, like a round ball. Every point on the surface is an equal distance from the center.

Formula for Calculating the Volume of a Sphere:

The volume \( V \) of a sphere can be calculated using the formula:

where:
- (pi) is a mathematical constant approximately equal to 3.14159.
- is the radius of the sphere.

What is a Hemisphere?

A hemisphere is half of a sphere, cut by a plane passing through the center, resulting in a flat circular face and a curved surface.

Formula for Calculating the Volume of a Hemisphere:

The volume  of a hemisphere is exactly half the volume of a full sphere, plus the volume of the flat circular face, which is negligible in the calculation of volume for solid bodies. Thus, the formula simplifies to:

Numerical Examples:

Example 1: Volume of a Sphere

Calculate the volume of a sphere with a radius of 6 cm. Using the sphere volume formula:

This calculation is essential for understanding volumes in tasks requiring precise spherical measurements.

Example 2: Volume of a Hemisphere

Calculate the volume of a hemisphere with a radius of 4 cm. Applying the hemisphere volume formula:

This example is particularly relevant in industries like culinary arts where hemispherical bowls are common.

Example 3: 

Imagine calculating the volume of a large decorative globe installed in a park with a radius of 10 feet. Using the sphere volume formula:

This example is useful in planning and constructing large-scale spherical installations.

Example 4: 

In landscaping, a hemisphere-shaped flower bed has a radius of 3 meters. Calculate its volume to estimate the soil needed:

This example helps landscapers and gardeners prepare adequate resources for plantings.

Example 5:

A luxury home includes a half-sphere swimming pool with a radius of 10 feet. Calculate the volume of water it can hold:

This example is useful in construction and maintenance planning for custom pools.

Real-Life Applications:

In Sports Equipment:

The design of balls used in sports like basketball, soccer, and others relies on understanding the volume of spheres for proper air pressure and bounce dynamics.

In Architectural Structures:

Domes and observatories often utilize the hemisphere shape, requiring accurate volume calculations for material estimation and structural integrity.

In Culinary Arts:

Chefs use hemspherical molds to create perfectly rounded dishes, where volume helps in recipe precision.

In Medicine:

Medical imaging techniques sometimes involve calculations of spherical volumes when assessing tumors or other rounded structures within the body.

Conclusion:

Mastering the volume calculations of spheres and hemispheres is not just academically beneficial but also immensely practical. This guide has provided the necessary formulas and examples to enhance your understanding and application of these geometric principles in various real-world contexts.

By exploring both the theory and practical applications of these volumes, students and professionals alike can apply this knowledge to solve real-life problems effectively and innovatively.