Volume of cylinders

Understanding Cylinder Volume: Essential Guide with Practical Examples:

Introduction to Volume Calculations for Cylinders:

Cylinders are among the most commonly encountered three-dimensional shapes in both academic studies and everyday life. From industrial drums to beverage cans, understanding how to calculate the volume of cylinders is a fundamental mathematical skill that is widely applicable. This blog post will guide you through the formulas needed to compute the volume of a cylinder and provide you with real-world numerical examples to solidify your understanding.

What is a Cylinder?

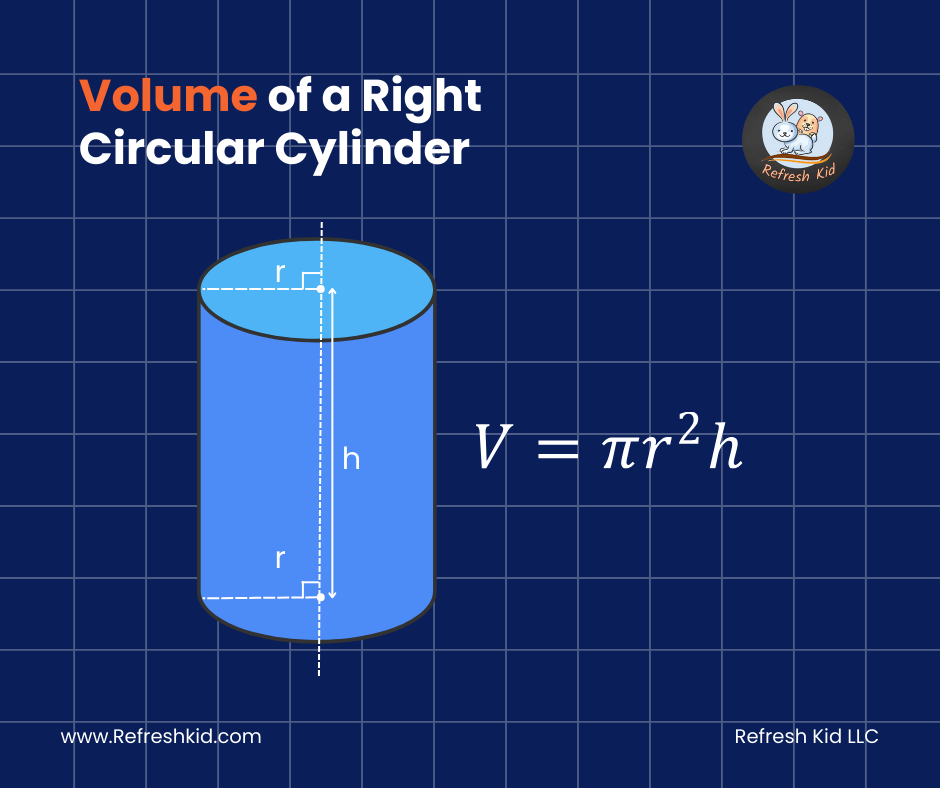
A cylinder is a three-dimensional shape with two parallel circular bases connected by a curved surface. The bases are always congruent and parallel to each other. There are two main types of cylinders: right cylinders, where the sides are perpendicular to the bases, and oblique cylinders, where the sides are at an angle.

Formula for Volume of a Cylinder:

The volume of a cylinder can be calculated using the formula:

Where:
- is a constant (approximately 3.14159),
- is the radius of the base of the cylinder,
- is the height of the cylinder.

Numerical Examples:

Example 1: 

Consider a simple right cylinder with a radius of 3 cm and a height of 10 cm.

Using the formula:

This calculation results in approximately 282.74 cubic centimeters.

Example 2: 

A municipal water tower, modeled as a cylinder, has a radius of 8 meters and a height of 20 meters.

This calculates to about 4,018.74 cubic meters, indicating the water storage capacity of the tower.

Example 3: 

A cylindrical plant holder used for home decor has a radius of 10 cm and a height of 30 cm.

The volume here is approximately 9,424.78 cubic centimeters, which helps in determining how much soil can be placed in it.

Example 4: 

Divers use cylinders that are essential for containing the compressed air they breathe underwater. For a diving cylinder with a radius of 3 inches and a height of 25 inches:

This translates to how much air a diver can carry, critical for planning dive lengths and depths safely.

Example 5:

In the petroleum industry, barrels are a standard unit for measuring volumes of crude oil. A typical oil barrel has a height of 34.5 inches and a radius of 12 inches.

This converts to about 42 gallons per barrel, essential for inventory and trading in the oil sector.

Example 6:

Concrete form tubes are cylindrical molds used for setting posts or building footings. Suppose a construction project uses a form tube with a radius of 6 inches and a height of 48 inches.

This volume helps determine how much concrete is needed to fill the form, crucial for ensuring the right amount of materials are ordered and used.

Practical Examples of Cylinder Volume Calculations:

Example 1: Beverage Cans

Designing beverage cans requires precise volume calculations to ensure each can holds the correct amount of liquid. A typical soda can with a radius of 3 cm and a height of 12 cm holds about 339.12 cubic centimeters.

Example 2: Garden Hoses

For a garden hose with a 1 cm radius and 10 meters length, calculating the internal volume helps determine how much water it can carry, crucial for irrigation planning.

Example 3: Fuel Tanks

In automotive design, understanding the volume of cylindrical fuel tanks, like a motorcycle tank with a radius of 15 cm and height of 40 cm, is vital for designing efficient fuel systems.

Conclusion:

Understanding how to calculate the volume of cylinders is invaluable for students, educators, and professionals across various fields. Whether you're designing containers, engineering pipes, or simply helping a student with homework, knowing these calculations provides a strong foundation in geometry. With the examples provided, you can better grasp the practical applications of these formulas in everyday life and enhance your mathematical prowess. For more educational content and resources, keep exploring our website to further your knowledge in mathematics and beyond!