Surface Area of Spheres and Hemispheres

Mastering the Surface Area of Spheres and Hemispheres: A Comprehensive Guide with Numerical Examples:

Discover the fascinating world of geometry as we delve into the calculations of surface areas for spheres and hemispheres. This guide provides an easy-to-understand approach to mastering these important geometric concepts, complete with numerical examples to enhance your understanding. Whether you're a student, educator, or professional, this article is your go-to resource for sphere and hemisphere surface area calculations.

Understanding Spheres and Hemispheres:

A sphere is a perfectly round three-dimensional object where every point on the surface is an equal distance from the center. Hemispheres are simply half of a sphere, split perfectly down the middle. These shapes are commonly seen in everyday life, from basketballs to planetary bodies.

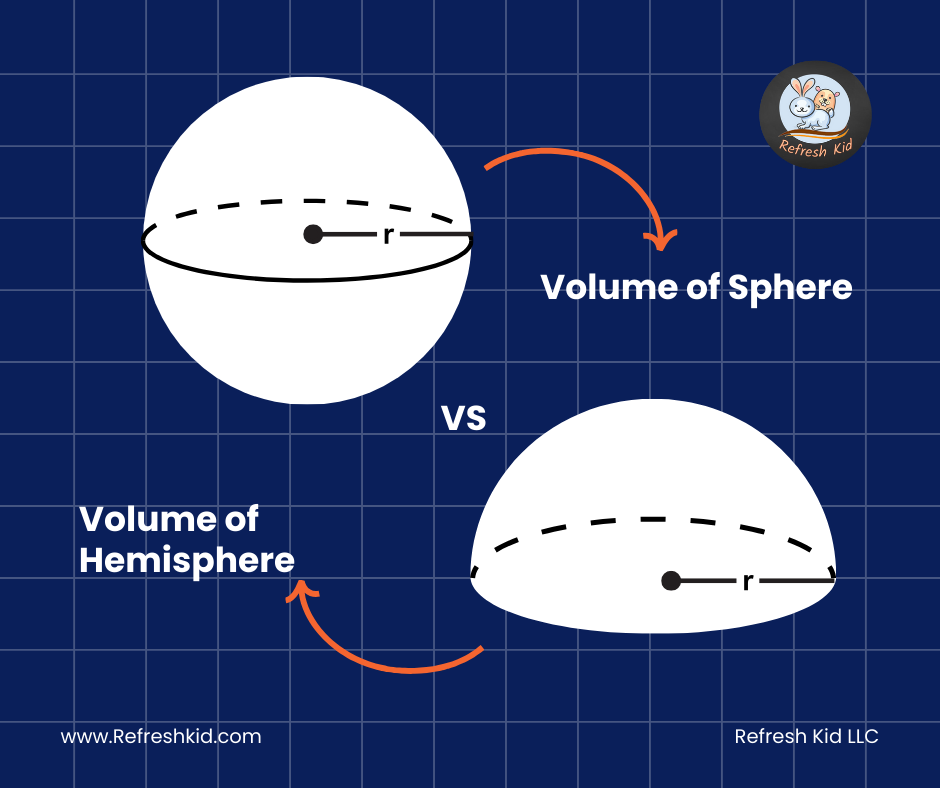
Surface Area of a Sphere:

The surface area of a sphere is crucial in various scientific and practical applications, such as calculating the material needed for a spherical tank or determining the exposure area in environmental studies.

Formula for Surface Area of a Sphere:

The formula to calculate the surface area A of a sphere with radius r is:

where is approximately 3.14159.

Example 1: Sphere

Suppose you want to paint a spherical balloon that has a radius of 7 cm. Using the formula above, calculate the surface area:

This calculation helps in determining how much paint you need to cover the balloon completely.

Surface Area of a Hemisphere:

Knowing the surface area of a hemisphere is useful for projects involving dome construction or when calculating the capacity and surface area of bowls and half-dome structures.

Formula for Surface Area of a Hemisphere:

The surface area A of a hemisphere includes the curved surface plus the base (a circle):

This formula considers for the curved surface and for the base area.

Example 2: Hemisphere

Imagine you need to wrap a decorative foil around a hemispherical dome with a radius of 5 cm. The surface area calculation would be:

This measurement is crucial for estimating the amount of foil needed.

More examples!

Example 3:

A miniature model of a planet, such as Earth, with a radius of 10 cm is used in a classroom setting. The surface area of this model can be calculated to help students understand the concept of scale:

Example 4:

A chef is designing a new chocolate bowl mold that is in the shape of a hemisphere. The radius of the mold is 8 cm. The total surface area is crucial for estimating how much chocolate is needed to make each bowl:

Example 5:

Consider a standard basketball, which typically has a radius of about 12 cm. To determine how much material is needed for the outer layer or to calculate the printing area for logos, calculate the surface area of the basketball using the sphere formula:

Practical Applications:

The knowledge of how to calculate the surface area of spheres and hemispheres has practical applications in numerous fields such as architecture, manufacturing, and environmental science. Accurate calculations are vital for efficient material usage and cost estimation.

Conclusion:

Understanding the surface area of spheres and hemispheres not only enhances your mathematical skills but also prepares you for real-world applications in various industries. With the detailed formulas and examples provided, you're now equipped to tackle any related problems with confidence.

Take advantage of this guide to expand your mathematical knowledge and apply these calculations in your academic or professional projects!