Surface area of Cones (Lateral and Total Surface Area)

Understanding the Surface Area of Cones: Lateral and Total Surface Area Explained with Numerical Examples:

Introduction to Surface Area of Cones:

Cones are a fundamental geometric shape used in both academic contexts and practical applications, such as architectural designs and everyday objects. This comprehensive guide will explain how to calculate both the lateral and total surface area of cones, featuring five detailed mathematical examples to aid in understanding these calculations.

What is a Cone?

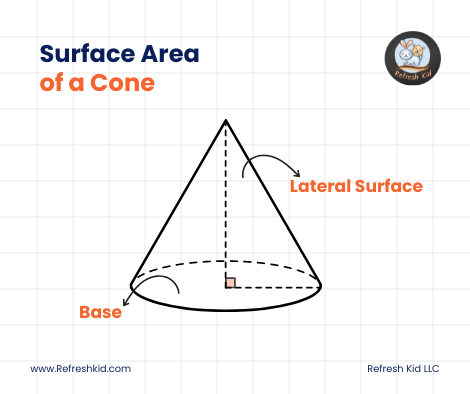
A cone is a three-dimensional geometric figure that has a circular base and a single vertex (apex) that is not necessarily directly over the center of the base, as in oblique cones, or may be right, as in right cones.

Lateral Surface Area of a Cone:

The lateral surface area (LSA) of a cone is the area of the cone’s surface excluding the base. It is useful for calculating the material needed for the conical part of an object.

Formula for Lateral Surface Area:

The LSA of a right circular cone can be calculated using the formula:

Where:

• is approximately 3.14159.
• is the radius of the circular base.
• is the slant height of the cone.

Mathematical Examples of Lateral Surface Area:

Example 1:

For a cone with a radius of 3 cm and a slant height of 5 cm:

This calculation gives approximately 47.12 cm².

Example 2:

For a cone with a radius of 4 cm and a slant height of 9 cm:

Approximately 113.1 cm².

Total Surface Area of a Cone:

The total surface area (TSA) of a cone includes both the lateral surface area and the base area. It is crucial for projects requiring material estimates for the entire surface.

Formula for Total Surface Area:

The TSA of a right circular cone is calculated by:

Mathematical Examples of Total Surface Area:

Example 3:

Using a cone with a radius of 4 cm and a slant height of 9 cm:

Resulting in approximately 163.36 cm².

Example 4:

For a cone with a radius of 5 cm and a slant height of 12 cm:

At=π×5×(5+12)=85π cm2

This totals approximately 266.76 cm².

Example 5:

Consider a cone with a radius of 7 cm and a slant height of 15 cm:

At=π×7×(7+15)=154π cm2

This results in about 483.56 cm².

Practical Applications:

These calculations are vital in many areas, including engineering for structural designs, in arts for creating realistic models, and in manufacturing for material estimation.

Conclusion:

Understanding how to compute both lateral and total surface area of cones is crucial for a wide range of scientific, educational, and practical applications. These five examples illustrate different scenarios to help reinforce your understanding and application of the formulas. Continue exploring our website for more resources on geometry and other mathematical topics to further enhance your knowledge and skills.