Exploring Special Segments in a Circle: Chords, Secants, and Theorems:

In the realm of geometry, circles hold a special place. Their symmetrical perfection and endless points of interest have fascinated mathematicians for centuries. One such aspect of circles that captures the imagination is the intricate interplay of segments that intersect within and outside the circle. In this exploration, we delve into two fundamental theorems governing these segments: the Segments of Chords Theorem and the Secant Segments Theorem.

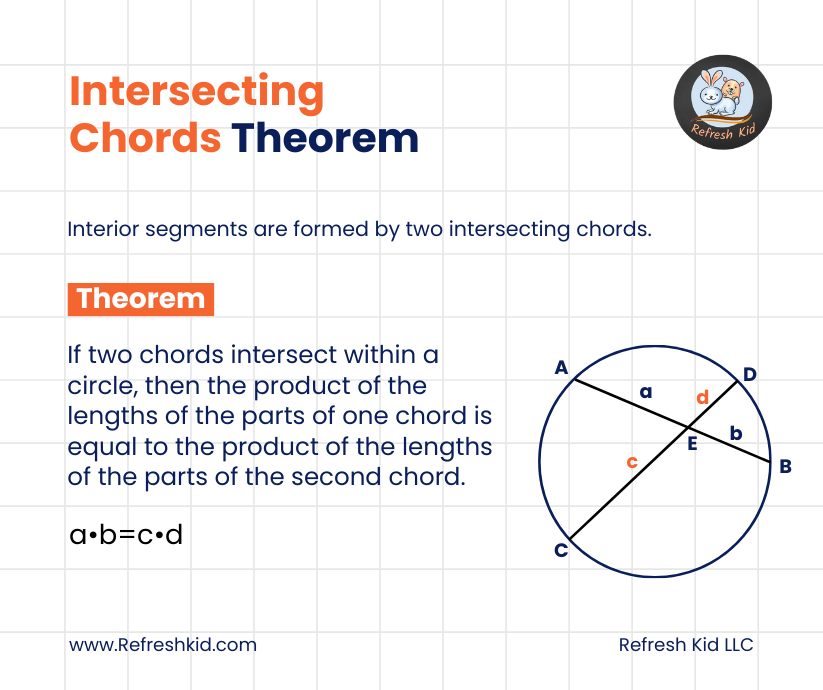
Segments of Chords Theorem: Unveiling the Mysteries Within:

Let's begin our journey within the confines of the circle, where chords intersect, creating fascinating segments. A chord, by definition, is a line segment whose endpoints lie on the circumference of the circle. When two chords intersect inside the circle, they divide each other into segments of various lengths.

The Theorem:

In a circle, if two chords intersect, then the product of the lengths of their respective segments is equal.

Mathematically, if $AB$ and $CD$ are two chords intersecting at point $P$ inside a circle, then:
$AP \times PB = CP \times PD$

Proof:

To prove this theorem, we can utilize similar triangles formed by the intersecting chords and the diameter of the circle. By applying the properties of similar triangles, we can establish the equality of the products of the segments.

This theorem not only unveils the elegant symmetry within a circle but also finds practical applications in various geometric problem-solving scenarios, from calculating distances to understanding the relationships between different parts of a circle.

Secant Segments Theorem: Bridging the Gap Outside:

As we venture beyond the circle's boundary, another intriguing phenomenon unfolds—the intersection of secants and tangents. A secant is a line that intersects a circle at two distinct points. When extended beyond those points of intersection, it creates fascinating segments both inside and outside the circle.

The Theorem:

In a circle, if a secant segment and a tangent segment intersect outside the circle at a point $P$ , then the square of the length of the secant segment is equal to the product of the length of the entire secant segment and its external segment.

Mathematically, if $AB$ is a secant segment, $AC$ is a tangent segment intersecting at point $P$ outside the circle, and $PD$ is the external segment of $AB$ , then:
$PA^2 = PB \times PD$

Proof:

The proof of the Secant Segments Theorem involves utilizing the power of a point outside a circle, which states that the product of the lengths of the two secant segments formed by an external point is equal to the square of the distance from the point to the circle's center.

This theorem not only enriches our understanding of the relationship between secants and tangents but also serves as a powerful tool in solving geometric problems involving circles, such as determining the lengths of segments or finding missing angles.

Conclusion: Navigating the Geometric Terrain:

In the vast landscape of geometry, circles stand as timeless symbols of symmetry and elegance. Within their confines lie a multitude of mysteries waiting to be unraveled, including the intricate interplay of segments formed by chords, secants, and tangents.

Through the Segments of Chords Theorem and the Secant Segments Theorem, we gain valuable insights into the relationships between these segments, both within and outside the circle. These theorems not only enrich our geometric toolkit but also deepen our appreciation for the beauty and complexity of mathematical structures.

As we continue to explore the wonders of geometry, let us marvel at the profound truths hidden within the simple yet profound confines of the circle, where lines intersect, segments divide, and the beauty of mathematics unfolds.