Law of Sines

Unlocking the Mysteries: Understanding the Law of Sines:

In the vast realm of trigonometry, certain principles stand out as fundamental pillars, guiding our understanding of triangles and their properties. One such principle is the Law of Sines, a powerful tool that illuminates the relationships between the sides and angles of any triangle. Join me on a journey as we unravel the mysteries of this fascinating law and explore its significance in mathematics and beyond.

The Essence of the Law of Sines:

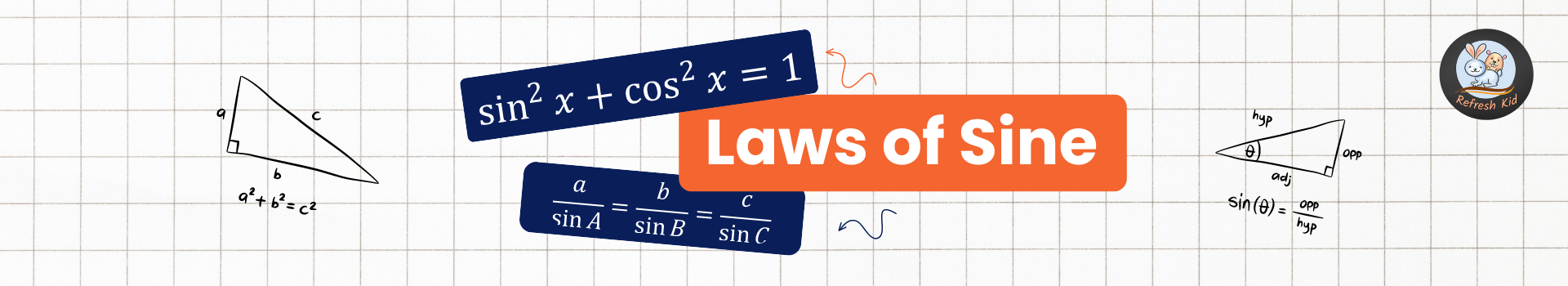
At its core, the Law of Sines establishes a relationship between the angles and the side lengths of a triangle. Specifically, it states that in any triangle, the ratio of the length of a side to the sine of its opposite angle is constant across all three sides:

Here, represent the lengths of the sides of the triangle, while denote the measures of the angles opposite those respective sides.

Visualizing the Law of Sines:

To better understand the Law of Sines, let's visualize its application in a triangle:

Consider a triangle ABC with side lengths a, b, and c, and opposite angles A, B, and C respectively. According to the Law of Sines:

This means that the ratio of the length of each side to the sine of the angle opposite that side remains constant across the triangle.

Example 1: Solving for a Missing Side Length

Consider a triangle where two side lengths and one angle are given. Use the law of sines to find the length of the third side.

Triangle ABC has side lengths AB = 5 units, BC = 7 units, and angle ∠B = 40 degrees. We want to find the length of side AC.

   Using the law of sines:
   
   

   We know:
 

   Solving for AC:
 

Example 2: Solving for a Missing Angle

Given a triangle where all three side lengths are known, use the law of sines to find one of the angles.

   Triangle XYZ has side lengths XY = 8 units, YZ = 10 units, and XZ = 12 units. We want to find the measure of angle ∠Y.

   Using the law of sines:
   
   

   Since we're interested in angle ∠Y, we focus on the ratio involving side YZ:
   
     Solving for ∠Y:
   
 

Example 3: Ambiguous Case

Sometimes, applying the law of sines can result in two possible solutions for a triangle. This occurs when given information could correspond to two different triangles. This situation is known as the "ambiguous case."
   
Consider a triangle where two sides and one non-included angle are given. The non-included angle is acute, and the given side is longer than the other given side. There could be one or two possible triangles, or no triangle at all, depending on the given data.
   
For instance, if you're given side lengths AB = 5 units, BC = 7 units, and angle ∠B = 30 degrees, you may find that there are two possible solutions for angle ∠A, leading to two possible triangles.

Applications in Real Life:

The Law of Sines finds numerous applications in various fields:

1. Navigation: Navigators and pilots use the Law of Sines to calculate distances and angles when plotting courses and determining positions, especially in celestial navigation.

2. Surveying: Surveyors employ the Law of Sines to measure distances and angles in land surveying, aiding in the creation of accurate maps and property boundaries.

3. Astronomy: Astronomers utilize the Law of Sines to calculate distances between celestial objects and determine the sizes and shapes of astronomical bodies.

4. Architecture and Engineering: Architects and engineers rely on trigonometric principles like the Law of Sines to design structures with precise angles and dimensions, ensuring stability and functionality.

5. Physics: Physicists apply the Law of Sines in various contexts, such as analyzing the motion of particles and understanding wave phenomena.

Challenges and Insights:

While the Law of Sines provides valuable insights into the relationships within triangles, it also presents challenges, particularly when dealing with ambiguous cases or non-standard triangles. Additionally, learners may encounter difficulties in applying the law correctly, especially when angles are not clearly defined or when side lengths are unknown.

However, mastering the Law of Sines opens doors to a deeper understanding of trigonometry and enables us to solve complex problems with elegance and precision.

Conclusion:

The Law of Sines stands as a cornerstone of trigonometry, offering a profound understanding of the relationships between angles and side lengths in triangles. From navigating the seas to exploring the cosmos, its applications are boundless, shaping our understanding of the world and empowering us to solve a myriad of real-world problems.

So, the next time you encounter a triangle, remember the guiding light of the Law of Sines, illuminating the path to deeper insights and discoveries in mathematics and beyond.