Unveiling the Mysteries of Arc Length and Sector Area in Circles:

Introduction:

Circles, with their perfectly symmetrical curves, have fascinated mathematicians for centuries. Within their round boundaries lie intriguing geometric properties waiting to be explored. Two such properties are arc length and sector area, which play fundamental roles in geometry and trigonometry. In this blog, we'll delve into the depths of arc length and sector area, uncovering their significance and providing insights into their calculations.

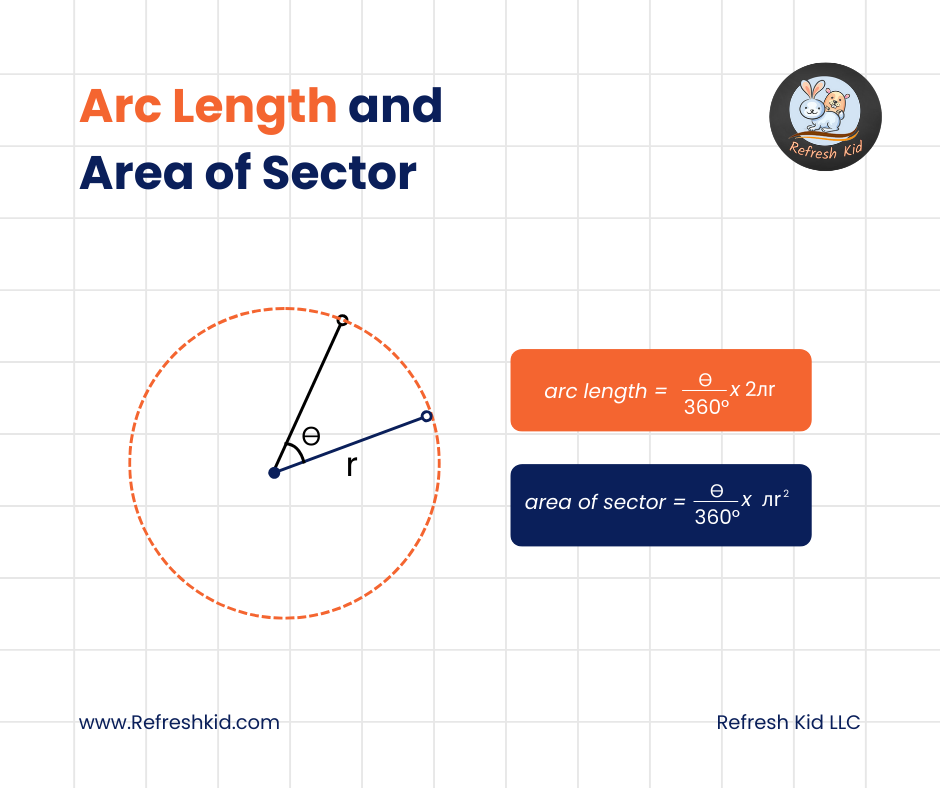
Understanding Arc Length:

Arc length refers to the distance along the curve of a circle between two points, typically measured in linear units such as centimeters or inches. It's analogous to the length of a piece of string wrapped around the circumference of the circle.

To calculate the arc length of a circle, we use a simple formula derived from the ratio of the angle subtended by the arc to the total angle around the center of the circle $(360 degrees or $2\pi$ radians)$ . This formula is given by:

$\text{Arc Length} = \frac{\theta}{360^\circ} \times 2\pi r$

or equivalently,

$\text{Arc Length} = \frac{\theta}{2\pi} \times 2\pi r$

where:
- $\theta$ is the measure of the central angle in degrees or radians.
- $\ r$ is the radius of the circle.

Understanding Sector Area:

A sector of a circle is a region bounded by two radii and the arc intercepted between them. Visualize cutting a pizza into slices; each slice represents a sector of the circular pizza. Just like arc length, the area of a sector can be calculated using the measure of the central angle.

The formula to find the area of a sector is derived from the ratio of the central angle to the total angle around the center of the circle. It's given by:

$\text{Sector Area} = \frac{\theta}{360^\circ} \times \pi r^2$

or equivalently,

$\text{Sector Area} = \frac{\theta}{2\pi} \times \pi r^2$

where:
- $\theta$ is the measure of the central angle in degrees or radians.
- $r$ is the radius of the circle.

Applications and Real-World Examples:

Understanding arc length and sector area is not just about solving mathematical problems; these concepts find practical applications in various fields. For example:
- In navigation, understanding the arc length of a circle helps in calculating distances between two points on the Earth's surface.
- In engineering and architecture, sector area calculations are crucial for designing curved structures and determining the amount of material needed.

Conclusion:

Arc length and sector area are fundamental concepts in geometry and trigonometry, offering insights into the relationships between angles, radii, and the curves of circles. By understanding these concepts and their formulas, mathematicians, engineers, and scientists can solve problems ranging from basic geometry exercises to real-world applications. So, the next time you admire the beauty of a circle, remember the mathematical intricacies that lie within its curves.