Unlocking Perspectives: Exploring Angles of Elevation and Depression:

In the vast landscape of mathematics, there are concepts that not only intrigue but also have practical applications in various fields. Among these is the captivating realm of angles of elevation and depression. Often overlooked in everyday conversations, these angles play a crucial role in fields ranging from architecture to trigonometry, providing us with valuable insights and tools to understand the world around us.

Understanding Angles of Elevation and Depression:

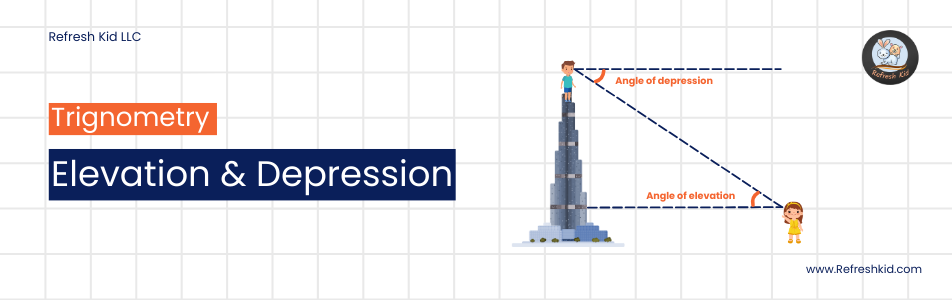
Imagine standing at a point and looking upwards or downwards towards an object. The angle formed between the line of sight and the horizontal level is what we call the angle of elevation or depression, depending on whether we are looking up or down, respectively.

To better visualize this, let’s consider a scenario: You're standing on the ground and observing an airplane flying overhead. The angle formed between the ground level and your line of sight to the airplane is the angle of elevation. Conversely, if you're looking down from a cliff at a boat sailing below, the angle formed between your line of sight and the horizontal ground is the angle of depression.

Applications in Real Life:

Angles of elevation and depression find numerous applications in real-life scenarios:

1. Architecture and Construction: Architects and engineers use these angles extensively to design structures such as bridges, buildings, and towers. Understanding angles of elevation and depression helps ensure proper alignment and stability of structures.

2. Surveying and Mapping: In cartography and surveying, precise measurements of angles of elevation and depression aid in creating accurate maps and charts, especially in mountainous or uneven terrains.

3. Navigation: Navigators, pilots, and astronomers use these angles to determine the position of celestial bodies, navigate aircraft, and plot routes.

4. Sports and Recreation: Angles of elevation and depression are also relevant in sports like golf, where players need to calculate the trajectory of the ball, as well as in activities like rock climbing, where climbers assess the incline of the terrain.

5. Physics: These angles are crucial in physics, particularly in projectile motion and optics, where understanding the trajectory of objects and the behavior of light is essential.

Mathematical Formulation:

Mathematically, angles of elevation and depression are often represented using trigonometric functions, particularly sine, cosine, and tangent. These functions relate the angles to the lengths of the sides of right-angled triangles, providing a means to calculate unknown angles or side lengths.

For example, if you know the height of an object and the distance from it, you can use trigonometry to find the angle of elevation. Conversely, if you know the angle of elevation and one side length of a right triangle, you can calculate other side lengths.

Challenges and Misconceptions:

Despite their importance, angles of elevation and depression can sometimes pose challenges for learners. One common misconception is the confusion between the two angles. Remember, an angle of elevation is formed when looking upwards from horizontal, while an angle of depression occurs when looking downwards.

Another challenge lies in visualizing and accurately measuring these angles in practical situations. This requires a solid understanding of trigonometric concepts and their applications.

Conclusion:

Angles of elevation and depression are not just abstract mathematical concepts; they are powerful tools that help us perceive and interact with the world around us. From designing structures to navigating through vast terrains, these angles shape our understanding of space and motion.

So, the next time you gaze at the stars, admire a skyscraper, or tee off on the golf course, remember the role that angles of elevation and depression play in shaping our experiences and knowledge of the world. Embrace these angles, for they provide us with a unique perspective on both mathematics and life itself.