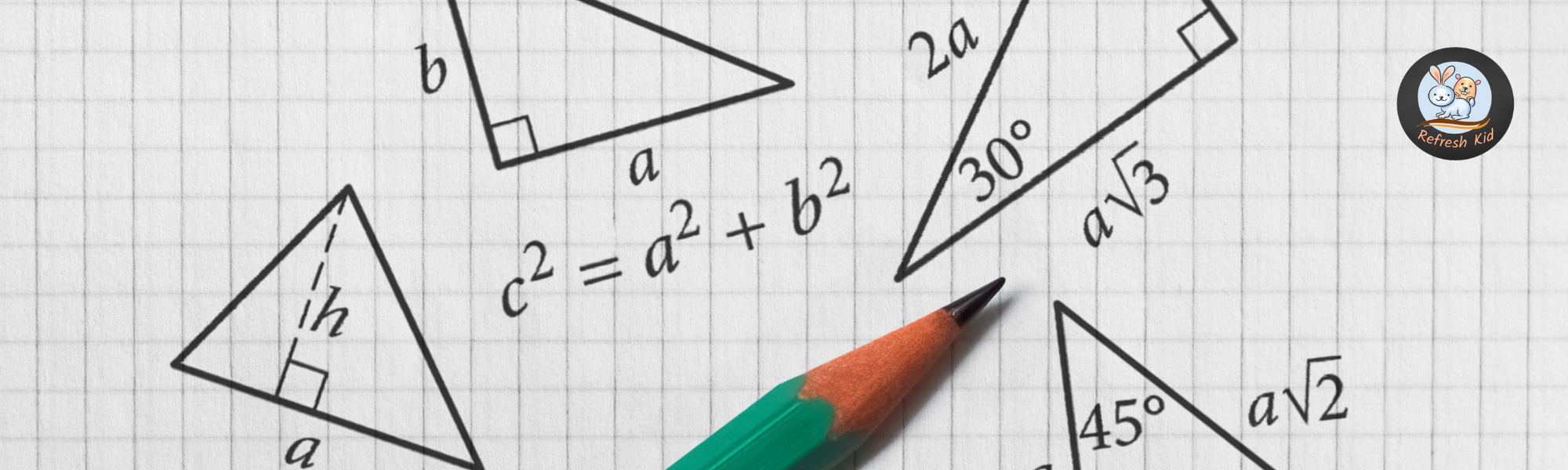The Pythagorean Theorem

Home
The Pythagorean Theorem

The Pythagorean Theorem

Mar 02 , 2024
By Refresh Kid LLC

Understanding the Pythagorean Theorem: A Beginner's Guide:

Introduction

Welcome to our comprehensive guide on the Pythagorean theorem, a fundamental concept in geometry that has applications in various fields, from architecture to astronomy. Whether you're a student, educator, or just a curious mind, this post will demystify the theorem for you in the simplest terms.

What is the Pythagorean Theorem?

The Pythagorean theorem is a principle in geometry, attributed to the ancient Greek mathematician Pythagoras. It relates to right-angled triangles, stating that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be expressed as $a^2+b^2=c^2$ , where $\large c$ is the length of the hypotenuse, and $\large a$ and $\large b$ are the lengths of the triangle's other two sides.

Formula:

$\large a^2+b^2=c^2 \text{ ;}$ Where $\large a$ is base, $\large b$ is height and $\large c$ is hypotenuse.

How to Use the Pythagorean Theorem:

Identify a Right-Angled Triangle: First, ensure your triangle has a 90-degree angle.
Label the Sides: Assign $a$ and bb to the two shorter sides and $c$ to the hypotenuse.
Apply the Formula: Use $a^2+b^2=c^2$ to find the missing side.

Real-Life Applications:

The Pythagorean Theorem isn't just for textbooks; it has practical applications in various fields:

Construction and Architecture: From designing buildings to laying out a garden, the theorem helps ensure structures are level and stable.
Navigation: It's used in navigation to calculate the shortest distance between points.
Art and Design: Artists use it to create compositions with proper perspective, making their paintings more lifelike.

Example 1: "The Perfect Picture Frame"

Imagine you've found a stunning picture frame at a flea market, shaped in a perfect right-angled triangle. The frame's base is 24 inches long, and its height is 18 inches. You're curious about the length of the diagonal side, which will help you determine the perfect spot on your wall.

Using the Pythagorean theorem, where the base is $\large a$ , the height is $\large b$ , and the diagonal side is $\large c$ , you can find out:

$a^2+b^2=c^2$

$24^2+18^2=c^2$

$576+324=c^2$

$900=c^2$

$c=\sqrt{900}=30$

So, the diagonal side of the frame is 30 inches long. You now know exactly how much space you'll need to hang your new treasure!

Example 2: "The Backyard Adventure"

You're planning a small garden in your backyard and want to set up a triangular patch for your vegetables. The two sides of the triangle you've planned out measure 40 feet and 30 feet. To buy the right amount of fencing, you need to know the length of the third side.

Applying the Pythagorean theorem:

$a^2+b^2=c^2$

$40^2+30^2=c^2$

$1600+900=c^2$

$2500=c^2$
$c=\sqrt{2500}=50$

You'll need 50 feet of fencing for the third side. Your garden planning just got a whole lot easier!

Example 3: "A Hiker's Dilemma"

You and your friend are on a hiking trail that leads straight up a hill, then turns sharply and continues straight to a lookout point. From the bottom of the hill to the turn is 300 meters, and from the turn to the lookout point is 400 meters. You're wondering what the straight-line distance from the bottom of the hill directly to the lookout point would be, cutting through the woods.

Using the Pythagorean theorem:

$a^2+b^2=c^2$

$300^2+400^2=c^2$