Master Pre-Algebra: The Gateway to Mathematical Triumph

Welcome to the fascinating world of Pre-Algebra! If you've ever wondered about the logical principles that underpin the language of numbers, equations, and patterns, you're in the right place. Pre-Algebra is the stepping stone to higher mathematical concepts, and mastering it sets the stage for your success in algebra and beyond. In this article, we'll take you on a journey through various aspects of Pre-Algebra, from the basic operations to the more complex equations. So, let's dive in and unlock the secrets of Pre-Algebra!

Pre-Algebra: What is it?  

Pre-Algebra is the bridge between arithmetic and algebra. It encompasses fundamental mathematical concepts and operations that prepare students to tackle more advanced algebraic equations and problem-solving techniques. Here are some essential topics covered in Pre-Algebra:

1. Arithmetic Operations in Pre-Algebra  

In Pre-Algebra, we build upon the four basic arithmetic operations: addition, subtraction, multiplication, and division. Understanding these operations is crucial as they form the backbone of mathematical calculations.

2. Integers: Embracing Positive and Negative Numbers  

Integers are whole numbers that can be either positive, negative, or zero. Learning to work with integers is a vital skill in Pre-Algebra, as it introduces students to the concept of directionality and the notion of negative quantities.

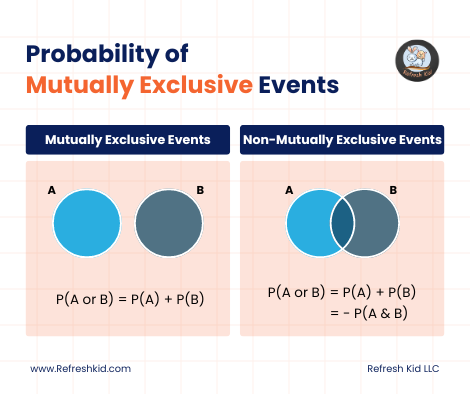
3. Solving Equations: Balancing the Scales  

Equations are mathematical statements that demonstrate equality between two expressions. Solving equations involves finding the value of the variable that makes the equation true. This skill is a foundational requirement for algebraic problem-solving.

4. Factors and Multiples: Unraveling Number Relationships  

Factors are numbers that divide evenly into a given number, while multiples are the results of multiplying a number by other integers. Mastering factors and multiples allows students to explore number relationships and understand divisors and products.

5. Fractions and Decimals: Dealing with Partial Values  

Fractions and decimals are essential components of Pre-Algebra, as they represent parts of a whole or values between integers. Understanding these concepts is vital for working with real-world measurements and calculations.

6. Proportions and Ratios: Relating Quantities  

Proportions and ratios describe the relationship between quantities, helping students compare and scale numbers in various contexts. These concepts play a crucial role in solving problems involving percentages and proportions.

7. Exponents and Powers: Understanding Repeated Multiplication  

Exponents and powers represent repeated multiplication of a base number, and they are fundamental in expressing large or small values more concisely. This concept is essential for advanced mathematical operations.

8. Functions: Building Mathematical Relationships  

Functions establish relationships between inputs and outputs. They are a fundamental concept in Pre-Algebra that lays the groundwork for understanding the more complex algebraic functions.

9. Graphing Basics: Plotting Points and Lines  

Graphing involves visually representing mathematical data on coordinate planes. Pre-Algebra introduces students to plotting points and drawing lines, which are essential skills for visualizing equations and data.

10. Inequalities: Comparing Quantities  

Inequalities express relationships between two values that are not equal. Learning about inequalities is vital for understanding ranges, intervals, and problem-solving involving inequalities.

11. Polynomials: Examining Expressions with Multiple Terms  

Polynomials are expressions containing two or more terms. Pre-Algebra introduces students to polynomials, preparing them for more complex algebraic manipulations.

12. Factoring: Breaking Down Expressions  

Factoring involves breaking down complex expressions into simpler terms. It is a critical skill in Pre-Algebra and is used extensively in algebra and calculus.

13. Pythagorean Theorem: Exploring Right Triangles  

The Pythagorean Theorem establishes the relationship between the sides of a right triangle. Pre-Algebra introduces students to this theorem, which has far-reaching applications in geometry and trigonometry.

14. Square Roots and Cube Roots: Finding Radical Solutions  

Square roots and cube roots involve finding the numbers that, when multiplied by themselves a certain number of times, yield a given value. Understanding these roots is essential for solving advanced equations.

15. Word Problems: Applying Pre-Algebra in Real-Life Scenarios  

Word problems challenge students to apply their Pre-Algebra skills in practical situations. They help bridge the gap between abstract mathematical concepts and their real-world applications.

FAQs  

Q: What are the prerequisites for learning Pre-Algebra?  

A: Pre-Algebra assumes a solid understanding of basic arithmetic operations such as addition, subtraction, multiplication, and division. It's recommended for students who have completed elementary arithmetic.

Q: Is Pre-Algebra necessary for advanced mathematics?  

A: Yes, Pre-Algebra serves as the foundation for higher-level mathematical subjects such as Algebra, Geometry, Trigonometry, and Calculus. A strong grasp of Pre-Algebra concepts is essential for success in these areas.

Q: How can I improve my problem-solving skills in Pre-Algebra?  

A: Practice is key! Engage in a variety of problem-solving exercises and work through different types of equations. Seek help from teachers or online resources if needed. you can take online tutoring at www.refreshkid.com.

Q: Are there any real-life applications of Pre-Algebra?  

A: Absolutely! Pre-Algebra concepts are used in fields such as engineering, physics, computer science, and finance. They are essential for solving real-world problems and making informed decisions.

Q: Can I learn Pre-Algebra on my own?  

A: Yes, you can. There are numerous online resources, textbooks, and tutorials available for self-study. However, having a dedicated teacher or tutor can provide valuable guidance and support.

Q: How can I stay motivated while learning Pre-Algebra?  

A: Set clear goals, track your progress, and celebrate your achievements. Join study groups or forums to connect with fellow learners and share experiences. Remember that mastering Pre-Algebra opens the door to exciting mathematical challenges.

Conclusion: Empowering Your Mathematical Journey  

Congratulations! You've completed your journey through the world of Pre-Algebra. We hope this article has ignited your passion for mathematics and inspired you to explore further. Remember, Pre-Algebra is not just about solving equations; it's about building a strong foundation for your mathematical success. Embrace the challenges, seek understanding, and let curiosity guide you as you continue your mathematical journey. Happy learning!